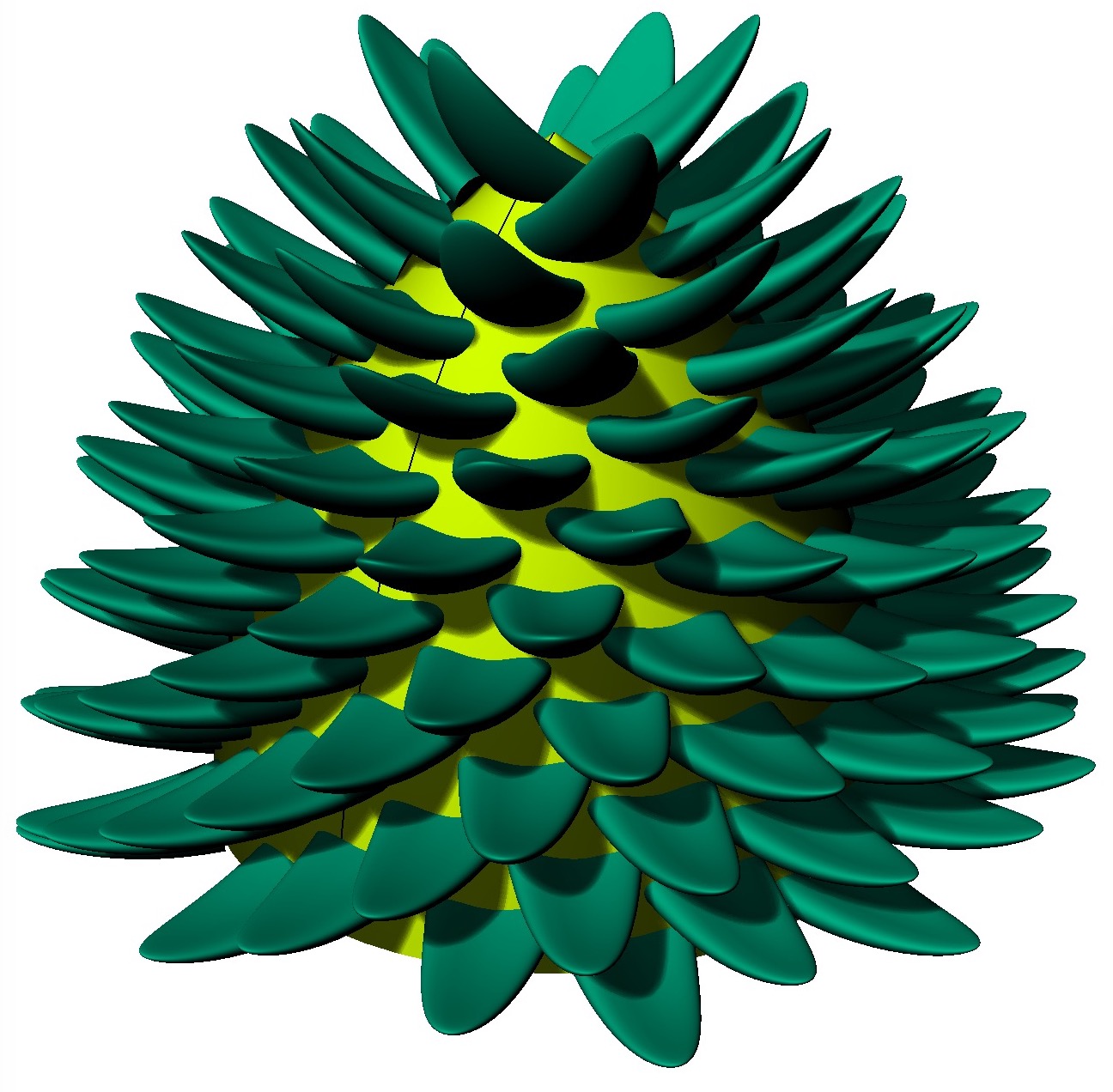
To say that a lot has been written about the arrangement of seeds on a sunflower would be a gross understatement. Discussions of this topic go back at least as far as D’Arcy Wentworth Thompson’s famous 1917 book, On Growth and Form. However, despite the wealth of available information, I’ve still found a few things
Category: Uncategorized
Back in October of 2016 I was contacted by Daniel Christev about an interesting modeling problem. It turned out that Daniel and I were mutual admirers of each others work. For anyone unfamiliar with his, I encourage you to check out his website at christevcreative.com. Daniel asked me if I had any thoughts about modeling a
Lot’s of exciting things have happened in the last few months, which unfortunately have led to a lot less blogging. Over the last few weeks I completely overhauled my website (davidbachmandesign.com), including pictures of all of my latest work, and a new online “store.” In November I was busy with my first (non-local) solo exhibition
At some point, perhaps in grade school, most people encounter the Mobius band: a simple shape made from a rectangular strip of paper by giving one end a half-twist before looping it around and gluing it to the other. The resulting surface has many interesting properties, both aesthetic and mathematical. Perhaps the most famous artistic image
In the next few posts I’ll briefly pause my discussion of organic modeling techniques, but I will come back to that soon (next up in the series will be on Diffusion Limited Aggregation… very cool stuff!). Today’s post is simply a brief announcement, just in case anyone missed all of my twitter, facebook, and forum posts
Organic Modeling Techniques Part 2: Differential Growth
Before I begin, a quick news update. My Grasshopper book is now completely through the copyediting process, and is with the printer. I’m told it will be available some time in the next few weeks! In the meantime, I’m happy to share the cover art. Now on to today’s post. As I said in my last post, my
Organic Modeling Techniques Part 1: Reaction-Diffusion
Before I get started with today’s post, I’m happy to announce the completion of my book, “Grasshopper: Visual Scripting for Rhinoceros 3D,” which is tentatively to be published March, 2017. This a basic introduction on the use of Grasshopper for 3D modeling. I wrote it with the Rhino user in mind who would like to start
Vein Structures
In honor of Halloween, I thought I’d post some spooky pictures and discuss how they were made. But first, please take a minute to check out my newly redesigned website, which is up and running at http://davidbachmandesign.com. A special thank you goes out to my friend, Ben Benjamin, for his advice on the design. Anyone who is interested
3D scanning for fun and profit
Most of my posts so far have been about experiments or challenges in 3-dimensional design for 3D printing. However, it’s possible to get into 3D printing without knowing much about design software, if you have a 3D scanner. In theory, to create an object suitable for 3D printing you can just sculpt it by traditional methods
BOBs!
I’m back from a long hiatus… The second half of the summer was busy with travel, and the beginning of the semester is always a difficult time. Only now are things settling down, and I can (hopefully) get back to regular posting. In the last few months my design consulting business has really taken off, and I will